Summary
This page offers some rough estimates of the numbers of wild animals on Earth. Collectively, wild land vertebrates probably number between 1011 and 1014. Wild marine vertebrates number at least 1013 and perhaps a few orders of magnitude higher. Terrestrial and marine arthropods each probably number at least 1018.
Contents
Introduction
Attempts to assess the magnitude of global biodiversity have focused on estimating species richness. [...] The total number of individual organisms in the world[...] has been a largely ignored statistic.
From a sentiocentric rather than ecocentric perspective, the number of animals in the world is actually much more important information than the number of species. Of course, if we're pessimistic about the net welfare of most wild animals, we may prefer for there to be fewer total wild animals.
Summary table
Note: This table shows my own amateur estimates. For estimates done by experts and published in a scientific journal, see the Bar-On, Phillips, and Milo (2018) section below.
| Animal Type | World Population |
|---|---|
| Lab animals | less than 108 (counting only vertebrates) |
| Humans | 8 * 109 |
| Livestock (terrestrial vertebrate farm animals) | 2.4 * 1010 |
| Birds | ~1 * 1011 to 4 * 1011 |
| Mammals | 1011 to 1012 |
| Reptiles | 1011 to 1014 |
| Amphibians | 1011 to 1014 |
| Fish | 1013 to 1015 or more |
| Earthworms | 1014 to 1017 |
| Dust mites | 1014 to 1017 |
| Coral polyps | 1015 to 1018 |
| Terrestrial arthropods | 1017 to 1019 |
| Rotifers | similar numbers as terrestrial arthropods?? |
| Gastrotrichs | ~1019 (?) |
| Copepods | ~1018 (?) |
| Nematodes | 1020 to 1022 |
| Protozoa (not animals) | 1023 to 1025 (??) |
| Bacteria (not animals) | 5 * 1030 |
Explanation of the estimates
Lab animals
Wikipedia reports: "Accurate global figures for animal testing are difficult to obtain; it has been estimated that 100 million vertebrates are experimented on around the world every year,[70] [...] The Nuffield Council on Bioethics reports that global annual estimates range from 50 to 100 million animals. None of the figures include invertebrates such as shrimp and fruit flies.[72]"
Fraser and MacRae (2011) estimate that the total "census numbers" (i.e., instantaneous population) of (vertebrate) lab animals worldwide are less than 100 million (p. 582, Table 1). They explain regarding this figure: "The number given by Taylor et al (2008) is 115.3 million. This is the estimated number of animals used per year. Because many of the animals used in science are rodents that live for less than one year, the number alive at any given time will presumably be less than 100 million."
If we added on invertebrates used in research, the numbers would be higher.
Livestock
The world livestock population in 2007 totaled roughly 24 billion (ignoring fish, lobsters, bees, and so on). This figure is calculated in the following table, which uses numbers copied from an FAOSTAT database.
| World Livestock Populations | Stocks |
|---|---|
| Total | 24,312,162,736 |
| Chickens | 17,863,376,000 |
| Cattle | 1,357,183,587 |
| Ducks | 1,095,805,000 |
| Sheep | 1,086,881,528 |
| Pigs | 918,278,483 |
| Goats | 830,391,683 |
| Turkeys | 472,635,000 |
| Geese and guinea fowls | 343,375,000 |
| Buffaloes | 177,247,938 |
| Horses | 58,641,094 |
| Asses | 42,038,633 |
| Camels | 24,246,291 |
| Other Rodents | 17,425,000 |
| Mules | 11,840,918 |
| Other Camelids | 6,861,765 |
| Animals Live Nes | 5,934,816 |
 Here's one simple check of the above numbers. Many broiler chickens in the US are killed at 5-7 weeks of age. Say it's 6 weeks. That implies (52 weeks per year)/(6 weeks per generation) = 8.7 generations per year. Given that ~9.2 billion meat chickens were killed in the US per year in 2010, the population of broiler chickens at any given time should have been (9.2 billion)/8.7 = 1.1 billion. Another 0.5 billion chickens were killed for eggs, and assuming ~1 generation of egg-laying hens per year, the total population of broilers + hens would have been 1.1 billion + 0.5 billion = 1.6 billion. FAOSTAT's database reports that in 2010, the US chicken population was 1,956,000,000, i.e., roughly 2 billion. So these estimates seem pretty close.
Here's one simple check of the above numbers. Many broiler chickens in the US are killed at 5-7 weeks of age. Say it's 6 weeks. That implies (52 weeks per year)/(6 weeks per generation) = 8.7 generations per year. Given that ~9.2 billion meat chickens were killed in the US per year in 2010, the population of broiler chickens at any given time should have been (9.2 billion)/8.7 = 1.1 billion. Another 0.5 billion chickens were killed for eggs, and assuming ~1 generation of egg-laying hens per year, the total population of broilers + hens would have been 1.1 billion + 0.5 billion = 1.6 billion. FAOSTAT's database reports that in 2010, the US chicken population was 1,956,000,000, i.e., roughly 2 billion. So these estimates seem pretty close.
The global numbers don't match as well. Over 65 billion land animals were killed for food globally around 2010, compared against 10.2 billion in the US in 2010. If the proportions of types of animals globally are the same as in the US, this would imply that (9.2 billion)/(10.2 billion) * (65 billion) = 59 billion of the global land animals killed were broiler chickens, and (0.5 billion)/(10.2 billion) * (65 billion) = 3.2 billion were laying hens. If there were 8.7 generations of broiler chickens per year globally, that would imply a population of (59 billion)/8.7 = 6.8 billion at any given time. Combined with 3.2 billion laying hens, that suggests 6.8 billion + 3.2 billion = 10 billion total chickens, when in fact, the number in the table above for 2007 was ~18 billion (and the proper comparison, the 2010 number from the database, was ~20 billion). Either this discrepancy is a symptom of bad data, or global proportions don't match US proportions, or chickens outside the US live longer. Maybe meat chickens in developing countries tend to live for several months before slaughter?
Noah Strycker estimates that there are 24 billion domestic chickens worldwide.
Birds
 Gaston and Blackburn (1997), in a paper titled "How many birds are there?", report: "we use a variety of methods to estimate the global number of individuals for a single taxon, birds. The different methods yield surprisingly consistent estimates of a global bird population of between 200 billion and 400 billion individuals" (p. 615). I think these numbers count all birds, not just breeding birds, based on two hints in the paper:
Gaston and Blackburn (1997), in a paper titled "How many birds are there?", report: "we use a variety of methods to estimate the global number of individuals for a single taxon, birds. The different methods yield surprisingly consistent estimates of a global bird population of between 200 billion and 400 billion individuals" (p. 615). I think these numbers count all birds, not just breeding birds, based on two hints in the paper:
- The authors write (p. 617): "Numbers fluctuate both through and between seasons. We ignore such details and seek order of magnitude estimates only. To some (unknown) extent fluctuations in different parts of the globe will be out of synchrony, so that fluctuations in the overall total number of individuals will be less than fluctuations in regional totals."
- Table 2 on p. 619 specifically flags some population estimates as being "breeding" birds.
In contrast, Gaston et al. (2003) estimated the total number of breeding birds specifically. The "typical" estimate for the global breeding-bird population in 1990 is 86.70 billion, with a range "between 39.34 and 134.04 billion individuals" (p. 1296), as shown in the screenshot of Table 1 below. Gaston et al. (2003) caution (p. 1295): "These figures are based on breeding birds, and would be inflated by non-breeders during the breeding season, and by post-breeding individuals at other times. Seabirds are ignored, as they contribute little to the overall total number of breeding birds [...]." So probably the population of all birds (breeding + non-breeding), averaged over each month of the year, is some small multiple of 86.70 billion. Hence, the lower bound of 200 billion from Gaston and Blackburn (1997) still seems to me potentially reasonable. (That said, these figures might also be biased a bit high because "Areas chosen for study may well[...] be biased towards higher avian densities; few ecologists intentionally pick ‘poor’ study sites" (p. 1295).)
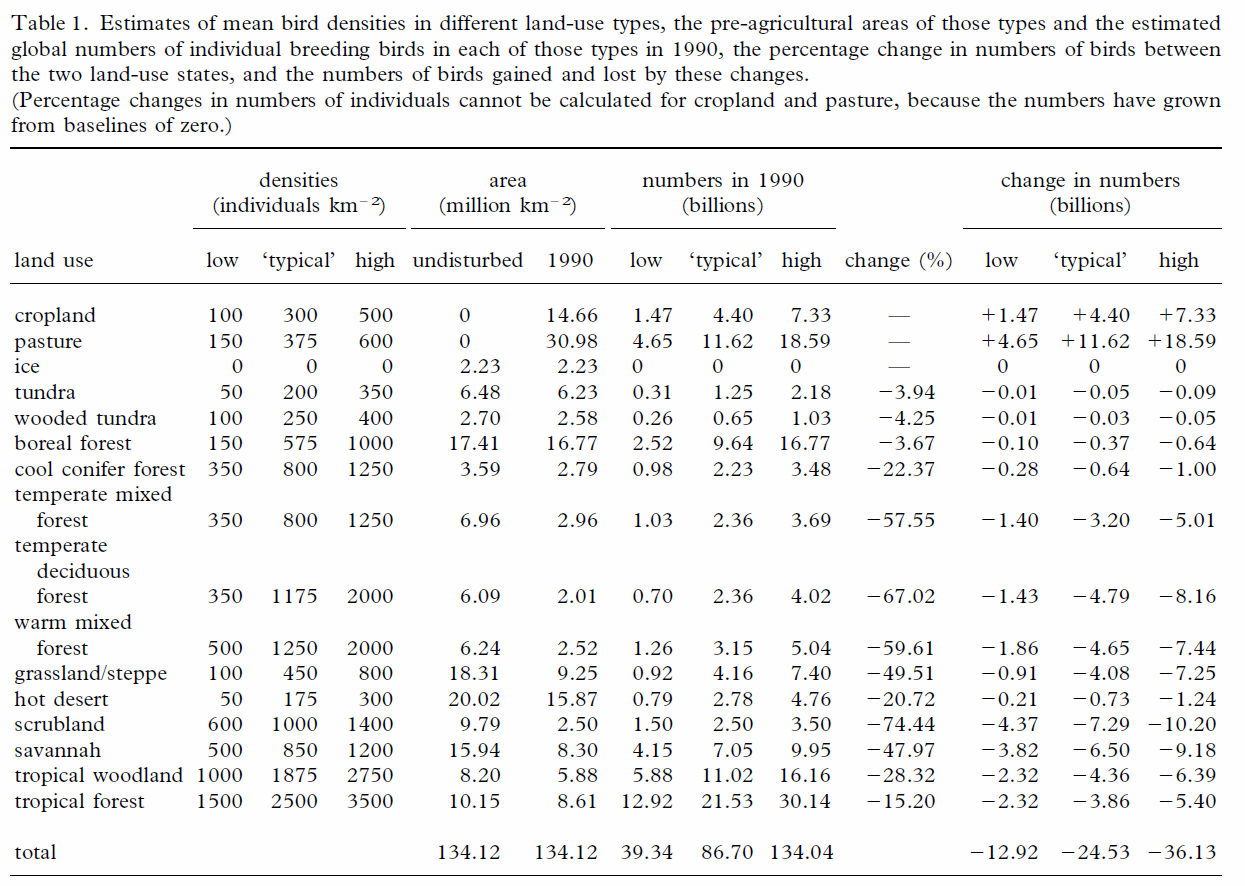
Gaston et al. (2003) also report (p. 1296):
Previous estimates put the total [number of birds] in the range 100–400 billion (Fisher & Paterson 1964; Wood 1982; de Juana 1992; Gaston & Blackburn 1997), but were based on unexplained methods or much cruder sets of data. Our calculations put the true figure at the lower end of this range.
This piece roughly agrees with the bird density for tropical forest specified above, since it says (p. 344) tropical rainforests have "a few dozen birds and mammals" per hectare. Two dozen birds per hectare is 2400 birds per km2.
Smil (2013) reports (p. 23) that biomass "for avifaunas usually do not surpass 0.05 g/m2 (Edmonds 1974; Reagan and Waide 1996)." Based on my reading of this page, it looks like backyard birds tend to have masses roughly in the range of 10 to 100 g. Then 0.05 g/m2 implies 10/0.05 = 200 m2 to 100/0.05 = 2000 m2 per bird, which implies a bird density of 500 to 5000 per km2. This seems reasonable in light of Gaston et al. (2003)'s Table 1.
Mammals
Matheny and Chan (2005), after discussing Gaston et al. (2003)'s bird estimates, report (p. 585) that "A similar review has not been conducted for mammals." So Matheny and Chan (2005) estimate a rough multiplier (p. 585):
Based on a British study by Gaston and Evans (2004) and Harris et al. (1995), here we assume the densities of wild mammals are 2.25 times those of wild birds for each land-use type. Applied to other continents, this is probably a significant underestimate, as Peters (1983, p. 167) records densities for some individual North American mammal species of over 10,000 individuals per square kilometer.
Multiplying a global bird population estimate of 100 to 400 billion by 2.25 gives an upper bound close to 1 trillion mammals.
A separately calculated estimate is based on Derek W. Yalden's article "A History of British Mammals". While noting the difficulty of estimating aggregate mammal populations, Yalden guesses a figure of 285 million wild mammals in Britain in the spring pre-breeding season, compared with ~48 million adult humans. (Yalden compares against Britain's adult human population rather than its entire human population "for fairer comparison with just the breeding populations of other mammals".) This implies 285/48 = 6 wild mammals for every human in Britain. In other countries, particularly those with less development and more rainforest, I would conjecture that the mammal-to-person ratio is higher. And then we need to add the mammals in the ocean. Overall, given ~1010 humans, it seems plausible there are at least 10 times as many wild mammals: ~1011. In fact, this ratio of ~10 wild mammals per human on Earth may be too conservative. Gaston and Blackburn (1997) estimate that there are roughly 40 to 60 birds per human worldwide, while "in Britain there are approximately 2 to 3 birds per head of human population" (p. 623).
Great Britain has a land area of 209,331 km2. So Yalden's figures suggest roughly (285 million wild mammals) / (209,331 km2) = ~1400 wild mammals per km2. Smil (2013) roughly agrees with this number (p. 21): "the expected density of animals weighing 10 g would be about 1,000/km2", where I assume that 10 g is intended to denote roughly the smallest classes of mammals.a It's not completely clear from Smil (2013)'s text, but I think the "1,000/km2" number may be a general approximation based on allometric scaling. Smil (2013) adds regarding this and other abundance estimates (pp. 21-22): "This would be only the best-fit values as the actual densities for every mass category range over at least two (and even three) orders of magnitude."
Smil (2013) also presents the following tableb of mammal biomass on p. 24:
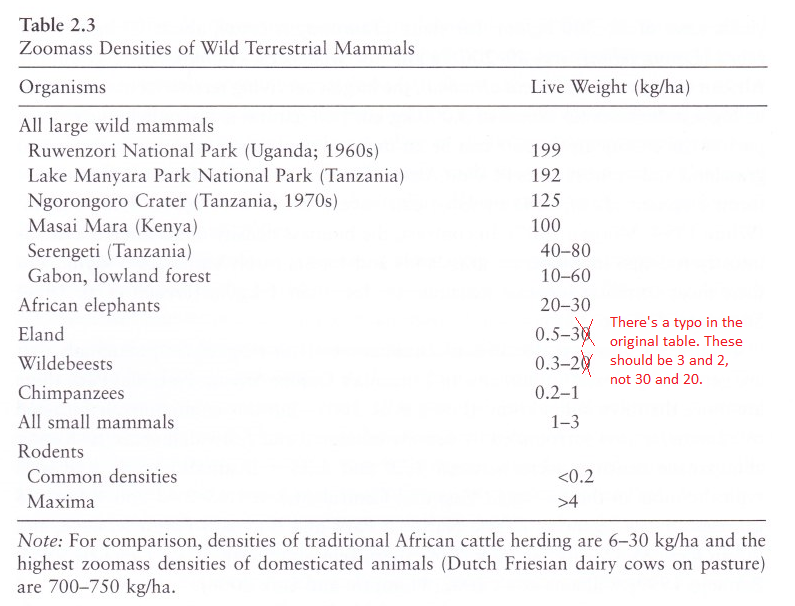
However, it's important to point out that the high zoomasses of large herbivores are reported in Africa. Smil (2013) says (p. 22) that "in Africa's savannas and rain forests [...] the mean mass of mammals is significantly greater than in Amazonia (Cristoffer and Peres 2003)." Therefore, "aggregate mammalian densities decline when expressed as averages for large regions or for biomes: at those scales, only savannas and some tropical forests harbor in excess of [20 kg/ha], while the mammalian zoomass in most equatorial and montane rain forests as well as in temperate woodlands is below [10 kg/ha] (Prins and Reitsma 1989; Plumptre and Harris 1995)" (p. 23).
Smil (2013) also gives the following rough figures (I think expressed as live weight?) as inputs to "a liberal estimate of the total zoomass of wild terrestrial mammals at the beginning and end of the twentieth century" (p. 227): "1 kg/ha in croplands, 2 kg/ha in low-productivity ecosystems (in both cases dominated by rodents), and 5 kg/ha (dominated by large herbivores) in the richest grasslands and forests". It's not clear if these numbers are based on specific sources or if they're generic estimates that Smil (2013) is partly making up based on his experience. 2 kg/ha = 200,000 g/km2, which would imply ~20,000 mammals per km2 if the mammals are 10 g each. That does indeed sound like a "liberal" estimate, although including larger mammals in this calculation would make it more reasonable.
Smil (2013) estimates (p. 227) "25 Mt of live weight [...] in the year 2000" for all wild mammals. 25 Mt = 25 * 1012 g, so my global wild-mammal population estimates of 1011 to 1012 imply 250 to 25 g per mammal. Of course, these numbers aren't very meaningful because much of this zoomass comes from large mammals.
Fittkau and Klinge (1973) suggest (Fig. 2, p. 8) the following biomass composition of mammals for "a central Amazonian rain forest near Manaus, Brazil" (p. 2). I added common names in purple based on Wikipedia. You can see that rodent biomass comprises a much bigger share of the total than in Smil (2013)'s Table 2.3 above. (Perhaps Smil (2013) himself would agree with this, given that he says (p. 78): "in contrast to grasslands and open woodlands, hunting in tropical rain forests is much more difficult because most of the resident zoomass is relatively small[ and for other reasons].")

Fittkau and Klinge (1973) explain regarding their animal-biomass numbers (p. 7):
The values have been derived from our general observations over the last 10 years and from observations of others, but not from actual counting and weighing [...]. Excepted are: soil fauna, total fresh biomass (84 kg per hectare; Beck 1970, 1971), and certain arthropods and vertebrates which we collected in the biomass estimation plot.
Reptiles
 Ishwar, Chellam, and Kumar (2001) assessed reptile densities in the tropical-rainforest floor of the Kalakad-Mundanthurai Tiger Reserve. Examining 5 m x 5 m quadrats, the researchers found an average of 0.2559 reptiles per quadrat = 10,240 reptiles per km2 (p. 413).
Ishwar, Chellam, and Kumar (2001) assessed reptile densities in the tropical-rainforest floor of the Kalakad-Mundanthurai Tiger Reserve. Examining 5 m x 5 m quadrats, the researchers found an average of 0.2559 reptiles per quadrat = 10,240 reptiles per km2 (p. 413).
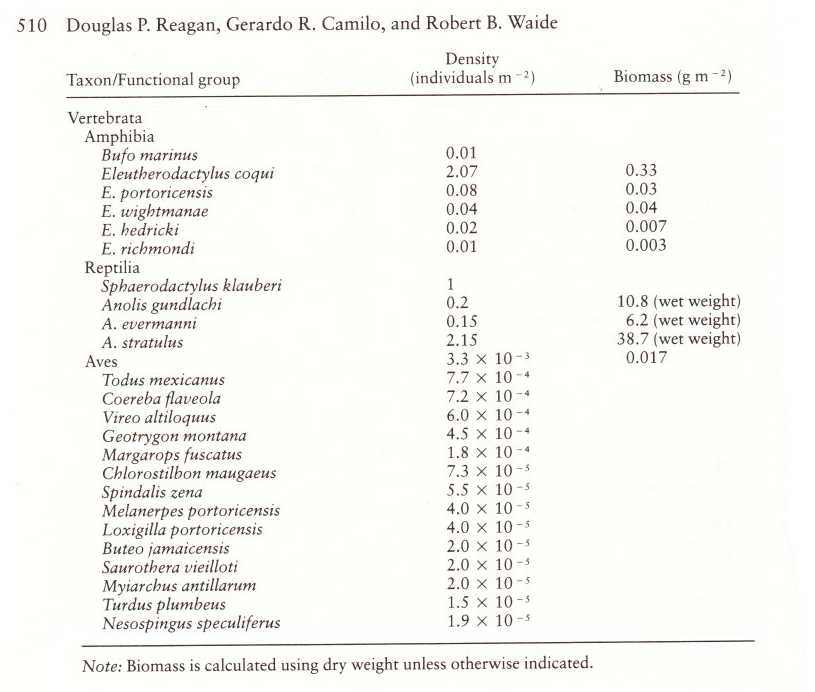
Reagan and Waide (1996) compiled research done on "a 40 ha area of forest around the El Verde Field Station in the Luquillo Experimental Forest of Puerto Rico" (pp. ix-x). Appendix 14.B (p. 510) reports the following vertebrate densities. Adding up the four reptile species gives 3.5 reptiles per m2 = 3.5 million per km2.
Looking at Table 1 from Gaston et al. (2003) above, we see that in 1990, the global area of tropical regions ("tropical woodland" + "tropical forest") was 5.88 million km2 + 8.61 million km2 = 14.49 million km2. Multiplying by ~104 to ~106 reptiles per km2 gives ~1011 to ~1013 reptiles in all tropical regions. This tropical land area is only (14.49 million km2) / (134.12 million km2) = 11% of the world's land area, but reptile densities are probably lower outside of the tropics. This book says (p. 28): "Being ecotherms, more lizard species live in the warm tropics than in temperate zones." So these estimates may be reasonable worldwide population estimates. Even if reptile densities per km2 outside the tropics were as high as in the tropics, the worldwide total number of reptiles would be only an order of magnitude higher.
Smil (2013), p. 21: "any large-scale averages of biomass densities of small ectothermic vertebrates (mainly frogs and snakes) are just statistical artifacts, as their densities are highly variable. But reptiles and amphibians can dominate the vertebrate zoomass in some tropical rain forests, where their overall [biomass] density may rival that of invertebrates (Reagan and Waide 1996)." Adding up reptile biomasses in the above Reagan and Waide (1996) table across the three species for which densities are reported gives 10.8 + 6.2 + 38.7 = 55.7 g/m2 = 557 kg/ha of wet weight.
Amphibians
Vasudevan, Kumar, and Chellam (2001) studied amphibians in the Kalakad-Mundanthurai Tiger Reserve. They found densities of roughly ~1 individual per quadrat = 40,000 per km2 (Fig. 2, p. 409).

Vasudevan, Kumar, Noon, and Chellam (2008), "Density and Diversity of Forest Floor Anurans in the Rain Forests of Southern Western Ghats, India," report frog-and-toad densities of 14,900 per km2 on the rainforest floor and over 30,000 per km2 near streams.
Huand and Hou (2004), "Density and Diversity of Litter Amphibians in a Monsoon Forest of Southern Taiwan ," identified between 35,000 and 102,400 amphibians per km2 (p. 798). They cite (p. 799) other studies that had assessed densities of both amphibians and lizards: Allmon (1991), which measured 23,000-155,000 amphibians and lizards per km2 in a South American rainforest, and Heatwole and Sexton (1966), Scott (1976), and Inger (1980), which found 75,000 to 360,000 individuals per km2 in Costa Rica and Panama.
The Reagan and Waide (1996) table in the "Reptiles" section above shows a total amphibian density, adding up each species, of 2.23 per m2 = 2.23 million per km2.
However, these trends may not extend to non-tropical biomes. One person wrote me the following comment: "Amphibians need to be close to water. [...] Quite a few birds in cold regions but not many amphibians." Sören and Carl expressed similar concerns about "extrapolating too much from studies in rainforests" regarding herpetofauna population numbers. For this reason, I'll stick to the conservative approach I used for reptiles of only calculating the number of tropical animals. Based on the above studies, there seem to be ~104 to ~106 amphibians per km2 of tropical forest. Multiplying this by a tropical area of 14.49 million km2 gives ~1011 to ~1013 amphibians. Being generous about amphibian populations outside tropical regions might push the upper bound toward 1014.
Fish
In another piece, I mention some estimates of the number of fish worldwide that fall between 1013 and 1015.
This article claims that bristlemouth fish alone may number 1014 to 1015.
This video explains that previous fish numerosity estimates had been ~3 * 1014, while newer estimates are ten times higher.
Earthworms
Brady (1974) reports (p. 118):
Because of their sensitivity to soil and other environmental factors, there is a wide variation in the numbers of earthworms in different soils. In very acid soils under conifers, an average of fewer than one organism per square meter is common. In contrast, more than 500 per square meter have been found on rich grassland soils. The numbers commonly found in arable soils is from 30 to 300 per square meter [...]. The biomass or wet weight for this number would range from perhaps 100 to 1,000 pounds per acre.
Based on the above, let's assume that the world average number of earthworms per m2 is between 1 and 1000 on biologically productive land (and zero elsewhere). Sundquist (2004) suggests that "87.35 million [km2] is something on the order of the amount of the world's reasonably biologically productive land." Rounding this to 100 million km2 implies 1014 m2.
Dust mites
Estimates of dust mites per person range from 105 to 107:
- According to "Dust Mite Allergy": "An average adult person may shed up to 1.5 grams of skin in a day, an amount that can feed one million dust mites!"
- Mayo Clinic reports 105 to 107 dust mites per bed.
- IndoorAir.com reports 107 mites per bed.
- National Geographic estimates 105 to 2 * 106 per bed.
(Do these numbers count only live dust mites? If they also include dead dust mites, the population of living dust mites would be lower.)
Dust mites are not present in all houses. This paper says "A 4-yr survey of mites in bedroom and family room carpets and couches in 252 homes of asthmatics located in 8 different geographical areas of the United States found that most homes (81.7%) were co-inhabited by both D. farinae and D. pteronyssinus (Arlian et al. 1992)." I'm uncertain how prevalent dust mites are in other parts of the world.
Dust mites may also be mostly absent (or at least quiescent) in colder, less humid months of the year or if home humidity is sufficiently low during summer months. This page says, "Well-ventilated homes in dry climates contain few dust mites." This paper echoes: "Field studies report that homes located in dry climates, such as those of the mountain states or upper Midwest, have few mites and little mite allergen present."
So a conservative estimate of the average number of dust mites per human on Earth might be several times lower than the lower bound of 105 dust mites per person mentioned above. Let's say the range is 104 to 107 dust mites per person. Multiplying this by ~1010 humans gives the range in the summary table at the top of this piece.
According to Wikipedia, female dust mites live at most 70 days and lay 60-100 eggs in the last 5 weeks of life. On average in a stable population, all but 2 of those offspring will die, perhaps painfully, before reproducing.
In Dust Mites (p. 83), Matthew Colloff reports that adult female Dermatophagoides pteronyssinus dust mites have brains 30-40 micrometers in diameter. Say it's 35 micrometers = .0035 cm. Then assuming a spherical brain, its brain volume is (4/3) * pi * radius3 = 2 * 10-8 cm3. For comparison a human brain's intracranial volume is 1700-1900 cm3. Of course, I would conjecture that mites have smaller neurons and vastly more efficient architectures per neuron than humans.
Fortunately, a few animal supporters seem to take dust mites seriously:
- "Will I crush dust mites when I sleep at night?"
- "Question: Do you crush dust mites when you walk or are they like ticks, you can step on them but it will have no effect?"
Sadly, I couldn't find answers to these questions. To be safe, I daily flap out my bed sheet into an unused area of my house in an effort to remove some of the dead skin on it. But if dust mites can be crushed, I may still be injuring tens of them each night? (Likewise when I put pressure on clothing, wash clothing, step on floor dust, throw dusty trash into a garbage compactor, etc.)
And if dust mites build up in pillows, is this an argument for replacing pillows regularly so that your head doesn't crush lots of mites? Or maybe most of the accumulated mites in pillows are dead?
This video shows dust mites under a microscope.
Face mites
As an aside, mites live not just in our beds but on our skin as well. I couldn't find authoritative data on face-mite populations, but here's one very rough attempt. One study took mite samples from six facial locations with cumulative surface area of 10 cm2 (p. 444). Mite counts on normal subjects averaged 10.8 individuals (Table I, p. 445). This suggests roughly 1 mite/cm2. I doubt the sampling sites were perfectly representative of all areas of the skin on the human head, but assume they were. Assume the human head is a sphere with radius ~10 cm. Its surface area is then 4 * pi * radius2 = 4 * pi * 102 = 1256 cm2, which implies ~103 mites per face and ~1013 mites added over all human faces. I don't know if this is about right or way off. By comparison with the bed dust-mite numbers above, it seems somewhat low, but maybe vastly more mites can live on the copious quantities of dead skin in beds than on the small amounts of dead skin and oils on people.
Coral polyps
One paper found coral polyp densities of 0.5-2 coral polyps per cm2. Since I haven't found other data on polyp densities, let's assume that the average density of polyps across all coral reefs is roughly the same as that: ~1 per cm2.
But is this density too low to be plausible? Polyps are typically only 1-3 millimeters in diameter, so it should be possible to fit several of them in a square centimeter. If polyps were 1 millimeter in diameter and stacked as tightly as possible, then it would be possible to fit 100 of them in a square centimeter. So in principle the density could be as much as 100 per cm2. More realistically, let's guess that it could be as much as, say, ~30 per cm2.
This implies ~1010 to ~3 * 1011 per km2. Note that a km2 of coral might occupy less than a km2 of land area because the coral has folds. So I'll guess that the density per km2 of flat land area is roughly between 1010 (lower bound) and 3 * 1012 (upper bound).
Coral reefs occupy an estimated 255,000 km2 of the Earth's surface, or about 3 * 105. That suggests a total population of coral polyps of roughly 3 * 1015 (lower bound) to 9 * 1017 (upper bound), which I rounded off as 1015 to 1018 in the summary table.
Note that the number of polyps should plausibly be less than the number of copepods, because polyps eat copepods (among other things). Also, because polyps are sessile, they probably have less developed nervous systems than most other animals.
Terrestrial arthropods
Many sources online report the number of insects as 1018 or 1019, without providing the details underlying this calculation. My guess is that these numbers may be based on an estimate by C.B. Williams. Williams (1960), p. 142:
To estimate the number of individual insects existing in the world at any given moment is an even more questionable occupation. In a series of careful extraction of insects from the soil at the Rothamsted Experimental Station in southeast England, the numbers in the top few inches of soil gradually rose, with improvements in technique, from 20 million to about 200 to 400 million insects per acre. One acre contains approximately 40 million square centimeters, so the indicated population was about 5 to 10 insects per square centimeter of surface. We do not yet have sufficient information about the population in tropical countries, or in hot and cold deserts; nor have we any accurate measurements of the number of insects that are normally above ground. Many places, however, must be more prolific than southeast England, and many less so. Since the land surface of the earth is not far from 50 million square miles, the number of square centimeters is of the order of 1.3 x 1018. On our British estimates we would therefore suggest about 8 x 1018 insects for the total. In view, however, of the great expanse of cold areas such as Greenland and the sub-arctic areas, this is possibly an over-estimate. It would seem that the total is unlikely to be less than 1017 or more than 1019. Let us therefore take 1018 as a working hypothesis.
I don't think Williams (1960) gives a citation for the Rothamsted Experimental Station measurements, so I'm not sure what taxa were included. Based on my reading of other reports of invertebrate densities in soils, such as Table 1 of Pearse (1946), an estimate of "about 200 to 400 million insects per acre" sounds to me like it probably includes mites and springtails, which, according to Pearse (1946)'s Table 1 as well as other authors, constitute most of the arthropods in soil on land. Mites are not technically insects. Springtails (formally known as Collembola) used to be considered insects but are no longer. I think Williams (1960) was counting springtails as insects because he says (p. 142): "It is probably amongst the smaller species, such as Collembola and aquatic insects such as midges, may-flies and mosquitoes that the really large numbers occur." (I thank Simon Knutsson for helping to make me aware of the point about springtails.)
As a result of the above discussion, I use the category "terrestrial arthropods" rather than just "insects" in order to include mites and springtails.
Gaston and Blackburn (1997) say (p. 617) regarding Williams (1960):
As far as we are aware, the only explicit attempt to calculate the overall number of individuals in a large taxon is that of Williams (1960). He estimated that the global insect fauna consists of around 1 x 1018 animals, based in part on the assumption that the global insect fauna is around three million species, which is probably unrealistically low.
In my reading of Williams (1960), I don't think the number of species played any role in his estimate of 1018 insects. The Williams (1960) passage quoted above is, as far as I can tell, the only basis for the 1018 estimate, and it isn't based on number of species. Maybe what Gaston and Blackburn (1997) had in mind was a more general sentiment like "People in 1960 underestimated the number of insect species, so maybe they also underestimated the number of insect individuals."
I think the Williams (1960) estimate is still pretty reasonable, and it agrees with what we might calculate based on other sources.
The Brady (1974) table here shows densities for "Other fauna" (i.e., non-nematode and non-earthworm invertebrate animals) in soils as 103 to 105 per m2. I assume this is mostly arthropods? As noted earlier, Sundquist (2004) suggests roughly 1014 m2 of biologically productive land area on Earth. This suggests 1017 to 1019 non-nematode and non-earthworm invertebrates on land, which is the same as the range suggested in the penultimate sentence of my above quote from Williams (1960).
Osman (2013): "Microarthropods mainly include mites and collembolans and are found in most types of soils. Hundreds of thousands of individuals [...] may be found within a square meter forest floor" (p. 116).
Rotifers
This page reports:
Population densities often reach greater than 1,000 individuals per liter. [... Rotifers represent] more than 50 % of the zooplankton production in some freshwater systems.
Rotifers may also be very abundant in the interstitial water of soils reaching densities up to 2 million per square meter.
This book agrees: "Depending on the soil type and its moisture level estimates of their densities range from about 32,000 to more than 2 million per square meter."
This book says that while rotifers are usually aquatic, "Rotifers may also be found in tens of thousands per square meter in unsaturated soils."
This book notes that rotifers are usually only found on land when the soil contains a significant amount of water films. One agricultural site in Costa Rica had tens of thousands of rotifers per m2. Rotifers "may reach numbers exceeding 105 per square meter in moist, organic soils (Wallwork, 1970)."
The estimates are variable, and I don't know how many hectares of soil has what density of rotifers. Rotifers are abundant in freshwater, though this covers only 1.77% of Earth's surface, compared with 30% for land. Until I get better numbers, I'll squint and say that rotifer densities seem maybe comparable to terrestrial-arthropod densities per square meter of soil, which suggests maybe similar abundances?
Gastrotrichs
This source includes the following table of gastrotrich density estimates (p. 166):
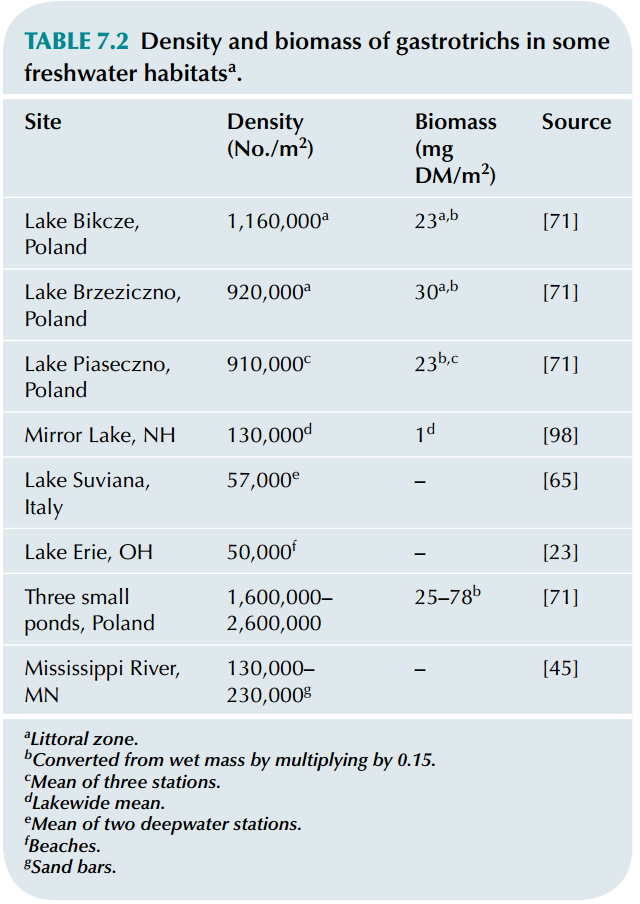
In case some of these estimates are biased high (because people will tend to study locations where the objects of study are more abundant), I'll use an estimate on the lower end: 50,000 gastrotrichs per m2 across all freshwater bodies.
This page reports that, excluding the Caspian Sea, "The world’s lakes have a combined surface area of about 5 million square kilometers".c That's 5 * 1012 m2. Assuming 50,000 gastrotrichs per m2, we have 2.5 * 1017 gastrotrichs worldwide in fresh water.
How about marine gastrotrichs? This page says:
In marine sediments, gastrotrich density may reach 364 individuals/10 cm2; typically they rank third in abundance following the Nematoda and the harpacticoid Copepoda, although in several instances they have been found to be first or the second most abundant meiofaunal taxon. In freshwater ecosystems population density may reach 158 ind/10 cm2 making the taxon rank among the top 5 most abundant groups.
The estimates in that quote are maximum densities, not average densities, so we can't draw immediate inferences about average densities. Still, it seems plausible that average marine densities per unit area are in a similar ballpark as average freshwater densities per unit area.
Earth's oceans cover about 360 million km2. Again assuming 50,000 gastrotrichs per m2, that implies (360 * 106 km2) * (106 m2 / km2) * (50,000 gastrotrichs / m2) = 1.8 * 1019. However, it's plausible this number is too high, since I'm unsure whether gastrotrichs can live throughout the oceans, including in the deepest parts??
Gastrotrichs can also live on land:
They inhabit the interstitial spaces between particles in marine and freshwater environments, the surfaces of aquatic plants and other submerged objects and the surface film of water surrounding soil particles on land.[4] They are also found in stagnant pools and anaerobic mud, where they thrive even in the presence of hydrogen sulphide.
I haven't counted terrestrial individuals, assuming they contribute less to the totals than marine individuals.
Copepods
Most zooplankton are copepods (p. 23). This source (p. 14) calls copepods "the most abundant animals in the ocean, possibly the most abundant on Earth" and estimates their population at 1018. This is consistent with a comment on p. 2 of the introduction to Insect Biodiversity Science and Society by Robert Foottit and Peter H. Adler, which explains: "The number of individual insects on earth at any given moment has been calculated at one quintillion (1018) (Williams 1964), an unimaginably large number on par with the number of copepods in the ocean (Schubel and Butman 1998) [...]."

Page 23 of this source reports on one study that found 3 million copepods per m3 of ocean water. If such a density held uniformly up to some depth d meters in the ocean all over the planet's 361.9 trillion m2 ocean surfaced (ignoring freshwater environments, where copepods reside as well), the number of copepods would be ~(1021) * d.
Plankton Safari also suggests a figure on the order of 1021 by assuming at least one copepod per liter in each of the 1.347 * 1021 liters of ocean water. However, in reality, I would guess there are few copepods in the very deep ocean and many more than one per liter near the surface.
Note that Antarctic krill numbers may also be quite high:
Planktonic copepods [...] are usually the dominant members of the zooplankton [...]. Some scientists say they form the largest animal biomass on earth.[11] Copepods compete for this title with Antarctic krill (Euphausia superba).
Nematodes
Nematodes have successfully adapted to nearly every ecosystem from marine (salt water) to fresh water, to soils, and from the polar regions to the tropics, as well as the highest to the lowest of elevations. They are ubiquitous in freshwater, marine, and terrestrial environments, where they often outnumber other animals in both individual and species counts, and are found in locations as diverse as mountains, deserts and oceanic trenches. They are found in every part of the earth's lithosphere, even at great depths (0.9–3.6 km) below the surface of the Earth in gold mines in South Africa. They represent 90% of all animals on the ocean floor. Their numerical dominance, often exceeding a million individuals per square meter and accounting for about 80% of all individual animals on earth, their diversity of life cycles, and their presence at various trophic levels point at an important role in many ecosystems.
Frazer (2013) affirms that "Four out of every five animals on Earth is a nematode." IowaPublicTelevision (2015) says the same: "four out of every five animals are nematodes".
Tomasik ("Abundances ...") presents sources that estimate nematode densities in terrestrial soils as generally between ~105 and ~107 per m2.
Petersen and Luxton (1982) (Fig. 4, p. 307) offer the following summary of the "Frequency distribution of mean density estimates" for nematodes in soil, based on a review of the literature. The Y axis shows "Number of estimates per density class. Each site or habitat is represented by one value only (viz. in some cases bi- or multiannual means)."
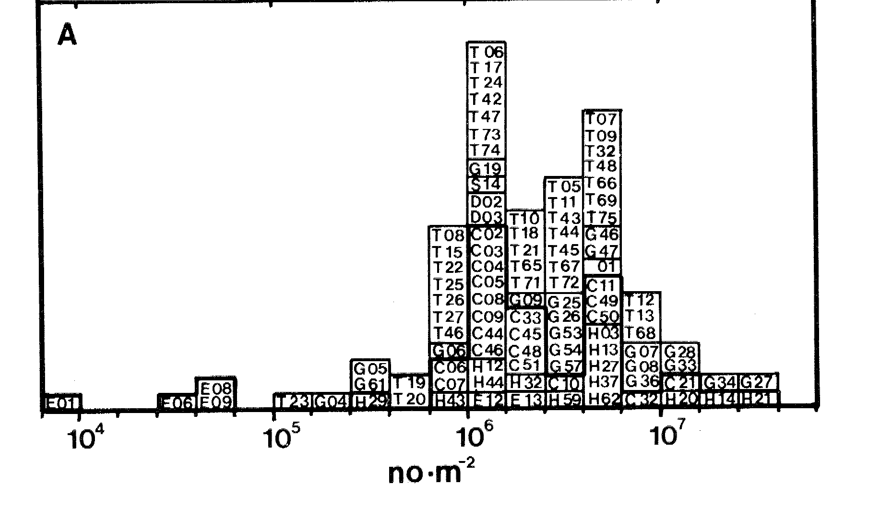
As noted earlier, Sundquist (2004) suggests roughly 1014 m2 of biologically productive land area on Earth. Assuming a range of ~105 to ~108 nematodes per m2 gives a total of 1019 to 1022 soil nematodes on land. I assume the number of marine nematodes is of a comparable order of magnitude.
A very thorough estimate of terrestrial nematode abundance is offered by van den Hoogen et al. (2019): "4.4 ± 0.64 × 1020 nematodes (with a total biomass of approximately 0.3 gigatonnes) inhabit surface soils across the world, with higher abundances in sub-Arctic regions (38% of total) than in temperate (24%) or tropical (21%) regions."
Ray (2017a): "Studies from the 1980’s and before suggest that there are several million nematodes per square meter of oceanic and terrestrial soil, which is an order of magnitude greater than other animals (Wharton & Surrey, 1994). This implies that there are about 2.5 x 1021 nematodes on earth’s surface".
Ray (2017b): "A global survey of marine benthic fauna looked at 128 studies at sites across the world. The study recorded benthic fauna abundance as it correlates with depth. Using this information and the amount of the Earth’s surface that occurs at various depths, I arrived at a number of 9.03E19 [i.e., 9.03 * 1019 ≈ 1020] microfauna on the ocean floor. These microfauna were mostly nematodes."
CSIRO (2009/2013) says "Nematodes are the most abundant and ubiquitous multicellular organisms on earth. [...] The total number of nematodes on earth is about 10 to the power 22". Unfortunately, no citation is given for this estimate.
It's unclear to me how much nematodes matter—certainly less than insects. Further reading: Nematode nervous system, C. elegans connectome.
Protozoa and bacteria
Protozoa (a somewhat outdated taxonomic group) are animal-like creatures that don't fall under the formal kingdom Animalia. We might extend very small amounts of ethical significance to these tiny creatures.
The Brady (1974) table here suggests that in soil, there are ~103 times as many protozoa as nematodes. Assuming this ratio holds true in non-soil environments as well, the world protozoan population would be about 103 times the world nematode population: 1023 to 1025??
The Brady (1974) table also shows bacteria as outnumbering protozoa by a multiple of 104, which gives an approximate world total of 1027 to 1029??
A more direct (and therefore more reliable) estimate of the number of bacteria in the world is 5 * 1030.
Biomass estimates
In this piece I've attempted to estimate abundance for various types of wild animals. Another metric besides counting the number of individual animals is biomass—the collective weight of animals or other life forms of a given type.
The Earth's Biosphere: Evolution, Dynamics, and Change by Vaclav Smil features an Appendix F on p. 284, "Estimates of the Biosphere's Heterotrophic Biomass" which can be viewed here. I reproduced the table below, changing Mt C to Gt C to make it easier to compare these numbers with those of Bar-On, Phillips, and Milo (2018) that I present later.
| Organisms | Biomass estimates (Gt C) |
|---|---|
| Prokaryotes | |
| Soils | 15 to 26 |
| Waters | 1.5 to 13.7 |
| Subterranean | 22 to 215 |
| Subsea | ? to 303 |
| Land | |
| Fungi | 3 to 6 |
| Invertebrates | 0.4 to 1 |
| Wild vertebrates | less than 0.005 |
| Elephants | 0.0001 |
| Domesticated vertebrates | 0.1 to 0.12 |
| Humans | 0.04 |
| Ocean | |
| Invertebrates | 0.3 to 0.5 |
| Fish | less than 0.04 |
| Whales | 0.005 to 0.015 |
Further explanation can be found in Smil's paper, "Harvesting the Biosphere: The Human Impact," and in Smil (2013). The results for land mammals specifically were made famous in an xkcd comic. Note that land invertebrates still outweigh humans 10-25 times according to these figures.
I'm skeptical of some of the numbers in "Estimates of the Biosphere's Heterotrophic Biomass". It lists fish as having a mass of less than 40 million metric tons of carbon, with whales adding another 5 to 15 million. Let's generously consider this as 40 + 15 = 55 million metric tons of carbon. Since carbon makes up 18.5% of the human body by mass, that translates to roughly 55/0.185 = 297 million metric tons in terms of wet mass. However, another paper found that the biomass of marine life bigger than 1 g in size was 197.97 + 176.03 + 156.51 + 139.16 + 123.73 = 793.4 million metric tons in terms of wet mass. For comparison, global catch from wild fisheries in 2005 was 93.2 million metric tons. Bar-On, Phillips, and Milo (2018) estimated the biomass of fish at 0.7 Gt C (see below), compared against Smil's "less than 0.04" Gt C.
Brain mass should correlate roughly linearly with overall biomass, given that brain-to-body-mass ratios typically don't differ by more than 1-2 orders of magnitude across species, so the biomass estimates may be a decent approximation of cumulative brain mass as well.
Counting total numbers of individual animals vs. counting total brain mass represent two relatively extremal positions for measuring the cumulative importance of animals. I think neither position is quite correct, and I would use some intermediate valuation, like maybe
(number of organisms) * sqrt(brain size per organism).
Let's approximate where my intermediate measure would leave us in comparing humans against, say, small mammals in terms of collective importance. Smil estimates (Table 2, p. 619) wild land mammals at 5 megatons of carbon, compared with 55 megatons for humans. Based on my "Summary table" at the beginning of this piece, I'll assume, say, 1012 land mammals and 1010 humans, i.e., 100 land mammals per human. Most of the land mammals are small, weighing at most (5/55)/100 ≈ 1/1000th of a human. This jibes with intuitive estimates: the average human weighs ~60 kg, and the average mouse weighs 20 to 40 g. The cumulative value of the small mammals using a sqrt(size) valuation is 1012 * sqrt(1/1000). The value of humans is 1010 * sqrt(1). The comparison reduces to 100 * sqrt(1/1000) vs. 1, i.e., ~3 to 1.
In general, if a group of N uniformly sized individuals collectively has mass M, the total importance of those minds, using the same formula, would be N individuals times sqrt(M/N) importance per individual, which equals sqrt(N*M).
My comparison between humans vs. small mammals ignores the fact that smaller, more r-selected animals probably suffer more per individual relative to their degree of sentience because, among other reasons, a (much) bigger percentage of their lives is composed of the agonizing experience of dying.
Bar-On, Phillips, and Milo (2018)
Bar-On, Phillips, and Milo (2018) and Bar-On, Phillips, and Milo (2018, "Supplementary") estimate the biomass and abundance of major biological taxa on Earth. The paper and its supplementary information are full of useful data, and I've only had a chance to skim a few parts so far.
Below, between two horizontal lines, I've reproduced the entirety of Table S1 (p. 89) from Bar-On, Phillips, and Milo (2018, "Supplementary").
Table S1. Summary of estimated total biomass and abundance of various abundant taxonomic groups.
Values are based on a literature survey as detailed in the SI Appendix. Reported values have been rounded to the closest order of magnitude in log scale thus reflecting the associated level of uncertainty.
| Taxon | Mass [Gt C = 1015 g C] | Abundance | |||
|---|---|---|---|---|---|
| Plants | Trees | 450 | 1013 | ||
| Bacteria | Terrestrial deep subsurface | 60 | 1030 | ||
| Marine deep subsurface | 7 | 1029 | |||
| Soil | 7 | 1029 | |||
| Marine | 1.3 | 1029 | |||
| Total | 70 | 1030 | |||
| Fungi | 12 | 1027 | |||
| Archaea | Terrestrial deep subsurface | 4 | 1029 | ||
| Marine deep subsurface | 3 | 1029 | |||
| Soil | 0.5 | 1028 | |||
| Marine | 0.3 | 1028 | |||
| Total | 7 | 1029 | |||
| Protists | 4 | 1027 | |||
| Animals | Chordates | Fish | 0.7 | 1015 | |
| Livestock | 0.1 | 1010 | |||
| Humans | 0.06 | 1010 | |||
| Wild mammals | 0.007 | ||||
| Wild birds | 0.002 | 1011 | |||
| Arthropods | Terrestrial | 0.2 | 1018 | ||
| Marine | 1 | 1020 | |||
| Annelids | 0.2 | 1018 | |||
| Molluscs | 0.2 | 1018 | |||
| Cnidarians | 0.1 | 1016 | |||
| Nematodes | 0.02 | 1021 | |||
| Total | 2 | 1021 | |||
| Viruses | 0.2 | 1031 | |||
These abundance values are comfortingly close to my own estimates. In particular, if we consider the ranges of abundance that I had in my "Summary table" at the top of this page before Bar-On, Phillips, and Milo (2018) was published, and compare against the point estimates of abundance in Bar-On, Phillips, and Milo (2018, "Supplementary")'s Table S1, then in cases where we were both making estimates for the same taxon, the Table S1 abundance value fell within the range in my "Summary table". The one exception was Protists, for which my range (1022 to 1025) was too low, although my range did almost capture the estimate of terrestrial protists of 3 * 1025 (Bar-On, Phillips, and Milo 2018, "Supplementary", p. 64).
This similarity in results is not too surprising given that Bar-On, Phillips, and Milo (2018, "Supplementary") used the same sources as I did for some of their abundance estimates: FAOSTAT for livestock, Gaston and Blackburn (1997) for birds, and Williams (1960) for terrestrial arthropods (Bar-On, Phillips, and Milo 2018, "Supplementary", pp. 62-63).
Biomass vs. respiration
One might conclude from the biomass numbers in Bar-On, Phillips, and Milo (2018, "Supplementary")'s Table S1 that plants are extremely dominant relative to other life forms, especially non-bacterial life forms. However, this is somewhat misleading, because most of the biomass in trees is biologically not very active. Nix (2018): "Only 1 percent of a dormant mature tree is biologically living while the rest is composed of non-living, structural wood cells."
Bar-On, Phillips, and Milo (2018, "Supplementary") estimate (pp. 6-7) that out of "450 Gt C of plant biomass", about "150 Gt C is concentrated in metabolically active plant tissues."
In my opinion, rather than biomass, a better proxy for the moral importance of a biological organism at a given moment is its respiration (Tomasik "Do ..."), since respiration measures active changes that are occurring. IPCC (n.d.) reports the following numbers related to global total respiration:
| Abbreviation | Definition | Amount (Gt C yr-1) |
|---|---|---|
| GPP | "Gross Primary Production denotes the total amount of carbon fixed in the process of photosynthesis by plants in an ecosystem" | 120 |
| NPP | "Net Primary Production denotes the net production of organic matter by plants in an ecosystem—that is, GPP reduced by losses resulting from the respiration of the plants (autotrophic respiration)." | 60 |
| NEP | "Net Ecosystem Production denotes the net accumulation of organic matter or carbon by an ecosystem; NEP is the difference between the rate of production of living organic matter (NPP) and the decomposition rate of dead organic matter (heterotrophic respiration, RH). Heterotrophic respiration includes losses by herbivory and the decomposition of organic debris by soil biota." | 10 |
Total autotrophic respiration is GPP - NPP = 120 - 60 = 60 Gt C yr-1, while total heterotrophic respiration is almost as high: NPP - NEP = 60 - 10 = 50 Gt C yr-1. (And this number presumably doesn't even count respiration by deep subsurface microbes.) From this perspective, plants no longer appear overwhelmingly dominant over everything else combined (though plants are still very important).
Bar-On, Phillips, and Milo (2018) report "that the total plant biomass (and, by proxy, the total biomass on Earth) has declined approximately twofold relative to its value before the start of human civilization." However, other authors have found that NPP has not declined nearly as much and may have actually increased due to humans (see Tomasik "Humanity's ..."). NPP is a much better proxy for total suffering than biomass (Tomasik "Net ..."), so we should avoid hasty conclusions from biomass numbers alone about how humanity has changed the rate of production of total suffering on Earth.
Skepticism about my mammal and bird numbers
Venkateshan K (2019) is an interesting blog post arguing that the numbers of wild mammals and birds that I reported in this piece may be inflated. A main reason to suspect this is that studies of animal abundance tend to be biased too high, because people often study areas where a given type of animal is most abundant. Trying to correct for this problem, Venkateshan K (2019) comes up with a potential estimate of 25.4 billion for the total number of wild mammals in the world and less than 2 billion for the number of wild birds. Venkateshan K (2019) acknowledges that these numbers, like mine, shouldn't be taken at face value, but that's part of his point. He says: "we should recognize the great uncertainty in our estimates of the relevant quantities in" the area of wild-animal suffering. I appreciate this critique, and I agree that estimates of wild-animal abundance contain considerable uncertainty.
Personally, I think wild invertebrates dominate the moral calculus in terms of the amount of total suffering on Earth today, followed perhaps by fish. Even without knowing the order of magnitude of their numerosity, it's clear that these types of animals far surpass farmed vertebrates in terms of number of individuals. (And they even outweigh farmed vertebrates by biomass, though by only about an order of magnitude.)
However, for someone who only cares about mammals and birds, drilling down on more precise estimates of wild-animal abundance appears important. I actually tend to think that if you only care about mammals and birds, you should probably focus on farm-animal advocacy, even if there are several times more wild mammals and birds than farmed mammals and birds. That's because farm-animal advocacy is more tractable, less likely to meet resistance from other compassionate people, and addresses some very severe forms of suffering. I think focusing on wild-animal suffering only begins to seem compelling once you move beyond mammals and birds, especially if you care about fish and invertebrates.
What are my thoughts on Venkateshan K (2019)'s calculation? I haven't dug into the details and am not a professional ecologist, but I would tentatively regard his calculation as one more data point out of a variety of data points we can consider. Any single fitted equation used to make estimates is unlikely to be super accurate.
Here's a gut check that makes me suspect the number of wild mammals is higher than 25.4 billion, although my method is far from scientific. As of Jul 2019 when I'm writing this paragraph, I live in a rural area of upstate New York, with various farms, fields, and forests nearby. Without even leaving my house, I can see a fair amount of wildlife from a window of my house looking toward the unmowed front lawn. Within the span of a few days in Jul 2019, I've seen at least the following mammals from the window: 1 adult rabbit, 1 immature rabbit, 1 woodchuck, and at least 1 chipmunk. (Update about two weeks later: I've now seen up to three rabbits simultaneously, as well as two chipmunks simultaneously.) In addition, there has recently (in the last few weeks) been at least 1 squirrel climing on the house. And there are usually numerous small rodents (mice? voles?) that run in the walls of the house at night. Just counting these mammals I can observe (either by sight or sound) suggests perhaps about ~10 mammals inside or close to my house. There may be many more small mammals around the house that never reveal themselves, and there must be several times this number in the wide expanses of fields and woods near my house. (Of course, many of the animals I observe from my window may also roam in the fields and woods. For example, I only see rabbits maybe 10% of the time when I look, so they must be grazing elsewhere most of the time.) If we include the unobserved nearby mammals, then even if a home typically contains several people, it still seems reasonable to estimate at least ~10 nearby wild mammals per person. For example, maybe there are 40 mammals near a 4-person rural household.
Not everyone lives in a rural area. World Bank (2018) estimates that 45% of people in the world were rural in 2018. The world population in 2018 was roughly 7.5 billion. 0.45 * (7.5 billion) = 3.4 billion. If we estimate that there are at least ~10 nearby wild mammals per person in a rural area, we would have a (very crude) lower bound of 10 * (3.4 billion) = 34 billion wild mammals. This doesn't even count areas of wilderness that are far away from human habitation. All told, my guess would be that the total would end up toward 100 billion or higher, but I don't know.
This estimate shouldn't be taken too seriously; it's just one more attempt to triangulate the true value. I tried this calculation based on animals around my house just because I have direct experience with them and I know that this location hasn't been cherrypicked to have abnormally high animal densities, which was a main concern expressed in Venkateshan K (2019).
See also
- Wikipedia's "Lists of organisms by population" has very incomplete population numbers.
- "What’s the most common animal species?"
Footnotes
- For example, a mouse has an average body weight of ~20 g. (back)
- As shown in red, there was a typo in two of the estimates, where the table said something different than Smil's text on p. 23. I think Smil's text, not his table, is correct, based on my skimming of Table 2 of Smil's source: Hayward, O’Brien, and Kerley (2007). There are a few other small typos in Smil (2013)'s book, such as
- writing "m2" instead of "m2" (p. 22)
- writing "after1850" instead of "after 1850" (p. 68).
These are helpful reminders that we shouldn't believe everything we read, even in published works by respected authors. (back)
- Sundquist (2004) reports a similar number: "A table in Section (se3-C) gives 4-6 million km2 of swamps, marshes, bogs, peat lands, lakes and streams." (back)
- In practice, plankton densities vary a lot throughout the ocean, so this assumption isn't valid. (back)